When it comes to maths, my students are capable of much more than I realised

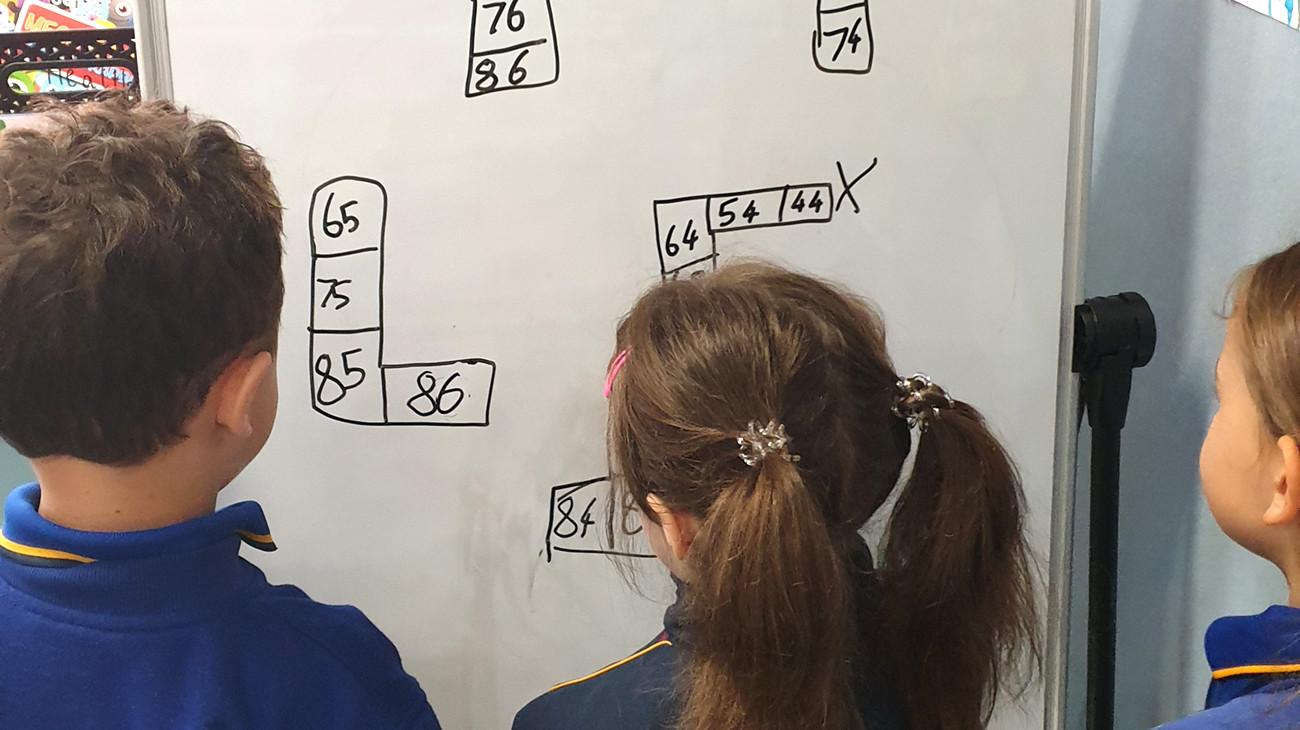
Hello and welcome to my first blog post! My name is Abi Cook and I teach a group of absolutely delightful Year 2 students at Settlers Primary School.

When I began my Alcoa Champions of Maths journey, I quickly realised that my idea of problem-solving was very different to what problem-solving actually is. My confidence in teaching maths in a way that allowed my students to discover, explore and reason was absent. I have been teaching the Year 2 class for four years and in that time, I had developed my teaching to be explicit, hands-on and engaging for my students but did not allow for them to independently apply solutions to calculate answers or discover answers in a way that was meaningful for them. Since starting this journey, my students have become huge lovers of problem-solving. Already, they are able to apply strategies, explain and justify their answers, listen to others and change their thinking.

For the first few problem-solving lessons my class did, they would branch off on their own. They seemed to lack the idea of working collaboratively and sharing their thoughts. They were competitive and would try and hide their answers from their group members so that no-one would “copy” their ideas. This has required an explicit teaching of the idea of group work and collaboration. And it’s still a work in progress. We celebrate groups that demonstrate group work and shared ideas. From this, they are learning that listening to their peers’ ideas is extremely valuable to their maths development.

Recently, I changed the way my students enter the explore phase of their problem-solving. Rather than sending them off into their groups and allowing them to begin recording ideas and answers straight away, they must discuss their ideas with their group for 2 minutes before they are given a marker. This not only allows them time to think about their own ideas, but also gives them time to listen to and question, agree or disagree with others. I have found this to be the most successful way of engaging my students in a productive discussion and allowing their maths thoughts to flow before recording.

One of the initial problem-solving tasks I did with my students was of adding and taking 10. I thought this would be a great concept for them to being looking at and understanding number patterns with the support of a hundreds chart because the students were in Term 1 and fresh out of Year 1. The problem was “I have an L shaped piece from a hundreds chart. On one of the squares of my piece is the number 65. What could the other numbers be?” I had some students who raised their hands straight away, ready to answer the question. I also had students who had no idea of the solution or how to get there. One of the reasons I find the Champions of Maths approach so effective is the mixed ability, random grouping which allows students of all ability levels to have a go, make mistakes and collaborate with their peers.

During the explore phase of this problem, I quickly noted that one of the misconceptions I had predicted was clearly evident in their working out. While moving around to each group, I asked them questions which guided their idea of counting by ones and by tens. One student told me “I put 65 here and counted around 66, 67, 68.” My response to their ideas was directing them to identify the pattern on the hundreds chart by saying “Have a look at the hundreds chart. Is that the pattern the numbers follow?” They were quickly able to realise their error and consequently, the rest of their solutions were correct. Students working within this group could also recognise that the numbers above 65 on a hundreds chart were decreasing and the numbers below 65 were increasing.

I find the discussion phase to be the most challenging yet most rewarding part of the lesson. The maths language and vocabulary that came out during the discussion in this lesson was brilliant. My students were able to correctly use the terms ‘increasing’ and ‘decreasing’ when sharing their ideas and answers with the class much more effectively. They could use the terms in context and even now, weeks after the lesson, they still refer back to increasing and decreasing on a hundreds chart. I asked students who were presenting “Why have you put 75 under 65?” and they gave me responses like “Because when you move down the hundred chart the numbers increase by 10” and “I put 75 under 65 because 75 is 10 more than 65 and each row increases by 10.” I also further pushed their thinking by asking them “If we add or subtract 10, which number changes?” They were not only able to recognise the vertical pattern of adding or subtracting 10 on the hundreds chart, but also the idea of place value and only the tens number changing when we add or subtract 10.

This lesson was very successful. After giving them an independent task to assess and consolidate their learning, I could see that 90% of my class really understood the idea of adding and subtracting 10. This was a huge reflection moment for me as a teacher, to understand and acknowledge that my students were much more capable of problem-solving than I had realised. I realised I could change the way I teach maths to be more effective for everyone in my classroom, including me. The way I approach maths now, particularly problem-solving, has altered drastically and I am so excited with the progress both my students and I have made. We are looking forwards to the rest of our ACoM journey this year.